The Biotic World is the best free Educational Website for Students. The Biotic World Provides NCERT Solution PDF, CBSE Notes PDF, NCERT Books PDF for class 8, 9, 10, 11, 12. The Biotic World also provides GU TDC Syllabus, Previous Years Question Papers, TDC notes on different topics of Physics, Chemistry, Mathematics, Zoology
and Botany any many more. So, Follow us to get tuned with latest updates.
Exercise 2 (a)
1. (i)  Or
Or 
Solution:
⇨Given
⇨
(ii) If a > 0, b > 0, a > b then 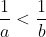
Solution:
⇨Given that
⇨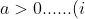)
⇨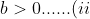)
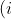\times(ii)\Rightarrow&space;ab%3E0)
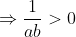
⇨Again

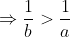
 Proved
Proved
⇨
⇨
⇨Again
2. (i) (\frac{1}{a}+\frac{1}{b})%3E4)
Solution:
⇨Adding 2ab On both side
(ii). (&space;\frac{1}{a}+\frac{1}{b}+\frac{1}{c})\geq&space;9)
Solution:
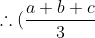(\frac{\frac{1}{a}+\frac{1}{b}+\frac{1}{c}}{3})\geq&space;(\sqrt[3]{abc})(\sqrt[3]{\frac{1}{abc}}))
(\frac{1}{a}+\frac{1}{b}+\frac{1}{c})}{9}\geq&space;\sqrt[3]{abc}.\frac{1}{\sqrt[3]{abc}})
(\frac{1}{a}+\frac{1}{b}+\frac{1}{c})}{9}\geq&space;1)
(\frac{1}{a}+\frac{1}{b}+\frac{1}{c})\geq&space;9) Proved
Proved
⇨If a, b, c>0 then Arithmetic Mean 
⇨If a, b, c>0 then Geometric Mean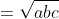
⇨Now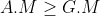
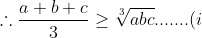)
)
⇨If a, b, c>0 then Geometric Mean
⇨Now
⇨Now (i) x (ii)
3.
Solution:
Solution:
Given





%3Ea(x+b))
%3Ea(b+x))
%3Ea)
 Proved
Proved
Given
Q.6. (i) If a > 1, b > 1 then (a+1)(b+1)>2(ab+1)
Solution:
Given
Similarly
Adding equation (i)+(ii)
Again
Dividing equation (iii) by (iv)
(ii) If a, b, c
Solution:
a, b, c > 0
We Know
Multiplying equation (i)(ii)(ii)
Q.7. (i)
Solution:
We know
Multiplying (a + b) on both side
(ii)
Solution:
Therefore
It means
Hence
Q.8.If a > b > 0 and c = 0 Then prove that
(i)
Solution:
Given
a > 0 , c > 0 = a + c < a .....(i)
b > 0 , c > 0 = b + c < b .....(i)
Dividing Equation (i) by (ii)
(ii)
Solution:
We know
Multiplying (a + b) on both side
(iii)
Solution:
We Know
Multiplying (a + b) on both side
(iv)
Q.9. If a, b, c, d are positive and
Solution:
Given
Adding
Therefore
Similarly
Adding
From equation (i) and (ii)
Q.10.If a, b, c are positive then show that
(i)
Solution:
Given a , b , c > 0
We Know
Similarly
Adding equation (i)+(ii)+(iii)
(ii)
Solution:
Given a , b , c > 0
We Know
Multiplying By c on both side
Similarly
Adding equation (i)+(ii)+(iii)
(iii)
Solution:
Given a , b , c > 0
We Know
Similarly
Adding equation (i)+(ii)+(iii)
Q. 10. If a > 0 , a