The Biotic World is the best free Educational Website for Students. The Biotic World Provides NCERT Solution PDF, CBSE Notes PDF, NCERT Books PDF for class 8, 9, 10, 11, 12. The Biotic World also provides GU TDC Syllabus, Previous Years Question Papers, TDC notes on different topics of Physics, Chemistry, Mathematics, Zoology
and Botany any many more. So, Follow us to get tuned with latest updates.
Exercise 9 (B)
1. Calculate the amount and the compound interest by using the formula for compound interest.
Q.N. |
Principal |
Rate Of interest |
Time (In years) |
1 |
Rs 625 |
4 % p.a. |
2 |
2 |
Rs 1000 |
10 % p.a. |
3 |
3 |
Rs 8000 |
10 % half-yearly |
1.5 |
Q.1.Solution:
⇨ Given p=625 r=4 % n=2
⇨Then the annual Compound interest will be
⇨ Total Amount = P + C.I. = 625 + 51 =676 Rs
Q.2.Solution:
⇨ Given p=1000 r=10 % n=3
⇨ Then the annual Compound interest will be
⇨ Total Amount = P + C.I. = 1000 + 331 =1331 Rs
Q.3.Solution:
^{2n}-1&space;\right&space;\})
⇨ Given p=8000 r=10 % n=1.5 or 3/2
⇨ Then the Half-yearly Compound interest will be
⇨ Total Amount = P + C.I. = 8000 + 1261 =9261 Rs
Q.4. Reena and Ruchira borrowed Rs 60000 and Rs 50000 respectively for a period of 3 years. Reena paid simple interest at the rate of 10 % p.a., while Ruchira paid compound interest at the rate of 10 % p.a. Compounded annually.Who paid more interest and by how much ?
Solution:
⇨For Reena
⇨Given
⇨p = 60000
⇨r = 10 %
⇨n = 3 p.a.
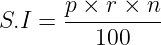
⇨Given
⇨p = 60000
⇨r = 10 %
⇨n = 3 p.a.
⇨Therefore the Simple interest for Reena will be
⇨Given
⇨p = 50000
⇨r = 10 %
⇨n = 3 p.a.
⇨Therefore the Compound interest for Ruchira will be
⇨From above Reena paid more interest than Ruchira by
= Interest paid by Reena - Interest paid by Ruchira
= 18000 - 16550 = 1450 Rs
Q.5. Sangeeta lent Rs 40960 to Amar to purchase a shop at 12.5 % per annum. If the interest is compounded semi-annually, find the interest paid by Amar after 1.5 or 3/2 years ?
Solution:
⇨Given
⇨p=40960
⇨r=12.5 %
⇨n=1.5 or 3/2
⇨Then semi-annually Then the semi-annually Compound interest paid by Amar will be
Q.6. Sudhir lent Rs 2000 at compound interest at 10 % payable yearly, While Prashant lent Rs 2000 at compound interest at 10 % payable half yearly. Find the difference in the interest received by Sudhir and Prashant at the end of one year?
Solution:
⇨In case of Sudhir
⇨Given
⇨p=2000
⇨r=10 %
⇨n=1 p.a.
⇨Then the annual Compound interest paid by Sudhir will be
⇨In case of Prashant
⇨Given
⇨p=2000
⇨r=10 %
⇨n=1 Half-yearly.
^{2n}-1&space;\right&space;\})
⇨Given
⇨p=2000
⇨r=10 %
⇨n=1 Half-yearly.
⇨Then the half-yearly Compound interest paid by Prashant will be
⇨Therefore The difference in the interest received by Sudhir and Prashant at the end of one year Will be
= 205 - 200
= 5 Rs
Q.7. Find the difference between simple interest and compound interest on Rs 16000 for 1.5 rears or 3/2 years at 5 % per annum ,compound interest being reckoned half yearly.
Solution:
⇨Given
⇨p=16000
⇨r=5 %
⇨n=1.5 annul for S.I.
⇨The simple interest will be
⇨Given
⇨p=16000
⇨r=5 %
⇨n=1.5 Half-yearly for C.I
⇨The compound interest will be
⇨p=16000
⇨r=5 %
⇨n=1.5 Half-yearly for C.I
⇨The compound interest will be
⇨Therefore the difference between S.I and C.I will be
= C.I - S.I.
=1230.25 - 1200
=30.25 Rs
=1230.25 - 1200
=30.25 Rs
Q.8. Find the compound interest on 3125 for 3 years if the rates of interest for first, second and third year are respectively 4%, 5%, 6%.
Solution:
⇨Given
⇨p=3125
⇨r1=4 %
⇨r2=5%
⇨r3=6%
⇨n=3 years
⇨For different rates on successive years, Total amount 'A' will be
Q.9. A District contains 64000 inhabitants. If the population increases at the rate of 2.5 or 5/2 % per annum ,find the number of inhabitants at the end of 3 years.
Solution:
⇨Given
⇨p=64000
⇨r=2.5 %
⇨n=3
⇨The number of inhabitants at the end of 3 years
Q.10. The nagar palika of certain city started campaign to kill the stray dogs which numbered 1250 in the city as a result, the population of stray dogs started decreasing at the rate of 20 % per month . Calculate the number of stray dogs in the city three months after the campaign started.
Solution:
⇨p=1250
⇨r=20%
⇨n=3 months
⇨The number of stray dogs in the city three months after the campaign
started Will be
^{n})
^{3})
^{3})
^{3})



 dogs
⇨Therefore no. of stray dogs=640
dogs
⇨Therefore no. of stray dogs=640
Q.11. The value of a machine depreciates each year by 10
% of it's value at the beginning of that year. It' value when
new is Rs 750, find it's value when it is 2 years old.
Solution:
⇨Given
⇨p=750
⇨r=10%
⇨n=2 months
⇨The value of a machine when it is 2 years old
will be
Q.12. The national wealth of a country increases by 4% of
it's value at the beginning of every year .Find the national
wealth of the country in 1985, if it was estimated as Rs 3.125
x 10^12 in 1983.
Solution:
⇨Given
⇨p=3.125 X 10^12
⇨r=4%
⇨n=2 years
⇨The national wealth of the country in 1984
⇨National wealth =3380 X 10^9 Rs
Q.13.The price of the scooter depreciates by 2% of it's at the
beginning of each year. Find The sale value of the scooter after 3
years , if it's present sale value is Rs 12000.
Solution:
⇨Given
⇨p=12000
⇨r=2%
⇨n=3 years
⇨The sale value of the scooter after 3 years will
be
⇨value of scooter=11294.304 Rs
Q.14. The population of Andhra Pradesh was 5.4 X 10^7 in
1988. If the population was growing at a constant rate of
2.4 % per annum .What was the population in 2008
A.D.[Given that (1.024)^20=1.60694].
Solution:
⇨Given
⇨p=5.4 X 10^7
⇨r=2.4%
⇨n=2008 - 1988 =20 years
⇨The population in 2008 will be
Multiple Choice Questions
Q.15. A steno- typist deposits Rs 10000 in a bank
for a period of 3 years at 12 % per annum compound
interest . The interest accrued to her after
maturity will be
Solution:
⇨Given
⇨p=10000
⇨r=12%
⇨n=3 years
⇨The interest accrued to her after maturity will be
Q.16. The present population of a city is 180000. If
it increases at the rate of 8% per annum , It's population
after 2 years will be
Solution:
⇨Given
⇨p=180000
⇨r=8%
⇨n=2 years
⇨Population after 2 years will be
High Order Thinking
Q.17. Umesh set up a small factory by investing ₹40,000. During
the first three successive years his profits were 5%,10%
and 15% respectively. If each year the profit was on
previous years' capital, calculate his total profit.
Solution:
⇨Given
⇨p=40000
⇨r1=5%
⇨r2=10%
⇨r3=15%
⇨Simple interest for 1st year will be



 Rs
⇨Total money invested in 2nd year=40000 + 2000 = 42000
Rs
⇨A/Q
⇨Profit in 2nd year =10%
⇨Then Simple interest for 2nd year will be
Rs
⇨Total money invested in 2nd year=40000 + 2000 = 42000
Rs
⇨A/Q
⇨Profit in 2nd year =10%
⇨Then Simple interest for 2nd year will be



 Rs
⇨Total money invested in 3rd year
= 42000 + 4200
= 46200 Rs
⇨Profit in 2nd year =15%
⇨Then Simple interest for 3rd year will be
Rs
⇨Total money invested in 3rd year
= 42000 + 4200
= 46200 Rs
⇨Profit in 2nd year =15%
⇨Then Simple interest for 3rd year will be



 Rs
⇨Total Money After 3 years
= 46200 + 6930
= 53130 Rs
⇨Therefore total profit will be
= 53130 - 40000
= 13130 Rs
Rs
⇨Total Money After 3 years
= 46200 + 6930
= 53130 Rs
⇨Therefore total profit will be
= 53130 - 40000
= 13130 Rs
⇨Given
⇨p=40000
⇨r1=5%
⇨r2=10%
⇨r3=15%
⇨Simple interest for 1st year will be
⇨Total money invested in 2nd year=40000 + 2000 = 42000
Rs
⇨A/Q
⇨Profit in 2nd year =10%
⇨Then Simple interest for 2nd year will be
⇨Total money invested in 3rd year
= 42000 + 4200
= 46200 Rs
⇨Profit in 2nd year =15%
⇨Then Simple interest for 3rd year will be
⇨Total Money After 3 years
= 46200 + 6930
= 53130 Rs
⇨Therefore total profit will be
= 53130 - 40000
= 13130 Rs
Q.18.The price value of the share of a company increase the
the rate of 15% in the first year suffered a loss of 10%
in the next year and again increased by 20% the third year
if the percent value of a share is Rs 2000 what will be
it's value after 3 years.
Solution:
⇨Given
⇨p=2000
⇨p=2000
⇨r1=15% increase
⇨r2=10% decrease
⇨r3=20% increase
⇨Simple interest after 1 year will be
⇨Share value after 1 year will be
= 2000 + 300
= 2300 Rs
⇨Simple interest after 2nd year will be
⇨Share value after 2nd year will be
= 2300 - 230
= 2070 Rs
⇨Simple interest after 3rd year will be
⇨Share value after 2nd year will be
= 2070 + 414
= 2484 Rs